Schrödinger Equation and Scatter Equation

February 17, 2011

GoLuckyRyan Basic, Scattering, theory , , Leave a comment

The Spatial part of the Time-Independent Schrödinger Equation (TISE) is

\left(- \frac {\hbar^2}{2m} \nabla^2 +V(r) \right) \psi(r) = E \psi(r)

by setting

k^2 = 2m E / \hbar^2   and    U(r) = 2 m V(r) /\hbar^2

the equation becomes a wave equation with a source, or scattering equation.

(\nabla^2 +k^2) \psi(r) = U(r) \psi(r)

for solving it, we have to find the Green function such that

(\nabla^2 + k^2 ) G(r,r') = \delta(x-x')

The solution is easy ( i will post it later, you can think the Green function is the inverse of the Operator)

G(r,r') = - \frac{1}{4\pi} \frac {Exp( \pm i k \cdot (r-r') )} { |r - r'| }

the particular solution is

\psi_p(r) = \int {G(r,r') U(r') \psi(r') dr'}

plus the homogeneous solution

\psi(r) = \phi(r) +\int {G(r,r') U(r') \psi(r') dr'}

but it is odd that the solution contain itself!

Optical Model

February 7, 2011

GoLuckyRyan advance, theory , , , , Leave a comment

In nuclear physics, the Optical Model means, we are treating the scattering problem is like optical wave problem. due to the incident beam can be treated as a wave-function. and this wave will be scattered by the target.

when the beam is far away from the target, the wave function of the incident beam should satisfy the Schrödinger equation in free space :

\left( \frac {\hbar^2 } {2m} \nabla^2 + V(r) \right) \psi( \vec{r} ) = E \psi ( \vec{r} )

and the plane wave solution is

\psi ( \vec{r} ) \sim Exp ( \pm i \vec{k} \cdot \vec {r} )

after the scattering, there will be some spherical wave come out. the spherical wave should also satisfy the free-space Schrödinger equation.

\psi( \vec{r} ) \sim Y(\theta, \phi) \frac {Exp( \pm i \vec{k} \cdot \vec{r} ) }{r}

Thus, the process of scattering can be think in this way:

Exp( \pm i k z ) \rightarrow Exp( i k z ) + f ( \theta ) \frac { Exp ( i k r ) } {r}

where f(θ) is a combination of spherical wave.

one consequence of using Optical Model is, we use complex potential to describe the nuclear potential terms in quantum mechanics.

when using a complex potential, the flux of the incident beam wave function can be non-zero. meanings that the particles in the beam are being absorbed or emitted. This corresponding to the inelastic scattering.

The reason for the “OPTICAL” is come form the permittivity and permeability of the EM field. for metallic matter, their permittivity or permeability may have a imaginary part. and this imaginary part corresponding to the absorption of the light. so, nuclear physics borrow the same idea.

the flux is defined as:

J = \frac { \hbar }{ 2 i m} ( \psi^*(r) \nabla \psi(r) - \psi(r) \nabla \psi^* (r) )

and the gradient of the flux, which is the absorption (sink) or emission ( source ) is:

\nabla J = \frac {\hbar }{ 2 i m }( \psi^* \nabla^2 \psi - \psi \nabla^2 \psi^* )

The Schrödinger equation gives the equation for the wave function:

\nabla^2 \psi(r) = \frac { 2m} {\hbar^2} ( E - V(r)) \psi(r)

when sub the Schrödinger equation in to the gradient of flux, we have:

\nabla J = \frac {1} {i \hbar } ( V(r) - V^*(r) ) | \psi |^2 = \frac { 2} {\hbar } Im ( V) | \psi |^2

we can see, if the source and the sink depend on the complex part of the potential. if the imaginary part is zero, the gradient of the flux is zero, and the wave function of the beam is conserved.

Larmor Precession (quick)

January 23, 2011

GoLuckyRyan Spherical Stuffs, theory , , , , , , , , , , , , , , , 1 Comment

Magnetic moment (\mu ) :

this is a magnet by angular momentum of charge or spin. its value is:

\mu = \gamma J

where J is angular momentum, and \gamma is the gyromagnetic rato

\gamma = g \mu_B

Notice that we are using natural unit.

the g is the g-factor is a dimensionless number, which reflect the environment of the spin, for orbital angular momentum, g = 1.

\mu_B is Bohr magneton, which is equal to

\mu_B = \frac {e} {2 m} for positron

since different particle has different mass, their Bohr magneton value are different. electron is the lightest particle, so, it has largest value on Bohr magneton.

Larmor frequency:

When applied a magnetic field on a magnetic moment, the field will cause the moment precess around the axis of the field. the precession frequency is called Larmor frequency.

the precession can be understood in classical way or QM way.

Classical way:

the change of angular momentum is equal to the applied torque. and the torque is equal to the magnetic moment  cross product with the magnetic field. when in classical frame, the angular momentum, magnetic moment, and magnetic field are ordinary vector.

\vec {\Gamma}= \frac { d \vec{J}}{dt} = \vec{\mu} \times \vec{B} = \gamma \vec {J} \times \vec{B}

solving gives the procession frequency is :

\omega = - \gamma B

the minus sign is very important, it indicated that the J is precessing by right hand rule when \omega >0 .

QM way:

The Tim dependent Schrödinger equation (TDSE) is :

i \frac {d}{d t} \left| \Psi\right> = H \left|\Psi\right>

H is the Hamiltonian, for the magnetic field is pointing along the z-axis.

H = -\mu \cdot B = - \gamma J\cdot B = -gamma B J_z = \omega J_z

the solution is

\left|\Psi(t) \right> = Exp( - i \omega t J_z) \left| \Psi(0) \right>

Thus, in QM point of view, the state does not “rotate” but only a phase change.

However, the rotation operator on z-axis is

R_z ( \theta ) = Exp( - i \frac {\theta}{\hbar} J_z )

Thus, the solution can be rewritten as:

\left|\Psi (t)\right> = R_z( \omega t) \left|\Psi(0)\right>

That makes great analogy on rotation on a real vector.

WKB approximation

January 11, 2011

GoLuckyRyan advance , , Leave a comment

I was scared by this term once before. ( the approach an explanation from J.J. Sakurai’s book is not so good)  in fact, don’t panic, it is easy. Let me explain.

i just copy what written in Introduction to Quantum Mechanics by David Griffiths (1995) Chapter 8.

The approx. can be applied when the potential is varies slowly compare the wavelength of the wave function. when it expressed in Exp( i k x) , wavelength = 2 π / k, when it expressed in Exp( - \kappa x ) , wavelength = 1/κ.

in general, the wavefunction can be expressed as amplitude and phase:

\Psi(x) = A(x)Exp(i \phi(x))

where A(x) and \phi(x) are real function

sub this into the time-independent Schrödinger equation (TISE)

\Psi '' (x) = - \frac {2 m} {\hbar^2 } ( E - V(x) ) \Psi (x)

\Psi ''(x) = ( A''(x)- A(x) \phi'(x)^2 + 2 i A'(x) \phi'(x)+ i A(x)\phi''(x) ) Exp(i \phi (x) )

and separate the imaginary part and real part.

The imaginary part is can be simplified as:

2 A'(x) \phi '(x) + A(x) \phi ''(x) = 0 = \frac {d}{dx} ( A^2(x) \phi '(x)

A(x) = \frac {const.} {\sqrt {\phi '(x)}}

The real part is

A''(x) = \left ( \phi ''(x) - \frac {2m}{\hbar^2 } ( E - V(x) ) \right) A(x)

we use the approx. that A''(x) = 0  ,  since it varies slowly.

Thus,

\phi '(x) = \sqrt { \frac {2m}{\hbar^2} (E - V(x) ) }

\Rightarrow \phi(x) = \int \sqrt { \frac {2m}{\hbar ^2} ( E - V(x ) )} dx

if we set,

p(x) = \sqrt { \frac {2m}{ \hbar^2 } ( E - V(x) )}

for clear display and p(x) is the energy different between energy and the potential. the solution is :

\Psi(x) = \frac {const.}{\sqrt {p(x)}} Exp \left( i \int p(x) dx \right)

Simple! but one thing should keep in mind that, the WKB approx is not OK when Energy = potential.

This tell you, the phase part of the wave function is equal the square of the area of the different of Energy and the Potential.

when the energy is smaller then the potential, than, the wavefunction is under decay.

one direct application of WKB approxi is on the Tunneling effect.

if the potential is large enough, so, the transmittance is dominated by the decay, Thus, the probability of the tunneling is equal to

Exp \left( - 2 \sqrt { \frac {2m}{\hbar ^2 } A_{area} ( V(x) - E )} \right)

Therefore, when we have an ugly potential, we can approx it by a rectangular potential with same area to give the similar estimation.

Hydrogen Atom (Bohr Model)

December 28, 2010

GoLuckyRyan atomic, Basic , , , , , , , Leave a comment

OK, here is a little off track. But that is what i were learning and learned. like to share in here. and understand the concept of hydrogen is very helpful to understand the nuclear, because many ideas in nuclear physics are borrow from it, like “shell”.

The interesting thing is about the energy level of Hydrogen atom. the most simple atomic system. it only contains a proton at the center, um.. almost center, and an electron moving around. well, this is the “picture”. the fact is, there is no “trajectory” or locus for the electron, so technically, it is hard to say it is moving!

why i suddenly do that is because, many text books said it is easy to calculate the energy level and spectrum for it. Moreover, many famous physicists said it is easy. like Feynman, Dirac, Landau, Pauli, etc… OK, lets check how easy it is.

anyway, we follow the usual say in every text book. we put the Coulomb potential in the Schrödinger equation, change the coordinate to spherical. that is better and easy for calculation because the coulomb potential is spherical symmetric. by that mean, the momentum operator (any one don’t know what is OPERATOR, the simplest explanation is : it is a function of function.) automatically separated into 2 parts : radial and angular part. The angular part can be so simple that it is the Spherical harmonic.

Thus the solution of the “wave function” of the electron, which is also the probability distribution of  the electron location, contains 2 parts as well. the radial part is not so trivial, but the angular part is so easy. and it is just Y(l,m) .

if we denote the angular momentum as L, and the z component of it is Lz, thus we have,

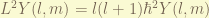
L^2 Y(l,m) = l(l+1) \hbar^2 Y(l,m)

L_z Y(l,m) = m \hbar Y(l,m)

as every quadratic operator, there are “ladder” operator for “up” and “down”.

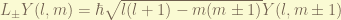
L_\pm Y(l,m) =\hbar \sqrt{l(l+1) - m(m\pm 1)} Y(l,m \pm 1)

which means, the UP operator is increase the z-component by 1, the constant there does not brother us.

it is truly easy to find out the exact form of the Y(l,m) by using the ladder operator. as we know, The z component of the a VECTOR must have some maximum. so, there exist an Y(l,m) such that

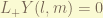
L_+ Y(l,m) =0

since there is no more higher z-component.

by solve this equation, we can find out the exact form of Y(l,m) and sub this in to L2, we can knowMax(m) = l . and apply the DOWN operator, we can fins out all Y(l,m) , and the normalization constant is easy to find by the normalization condition in spherical coordinate, the normalization factor is sin(\theta) , instead of 1 in rectangular coordinate.

\int_0^\pi \int_0^{2 \pi} Y^*(l',m') Y(l,m) sin(\theta) d\theta d \psi = \delta_{l' l} \delta_{m' m}

more on here