Magnetic Dipole Moment & Gyromagnetic Ratio

January 16, 2016

GoLuckyRyan Basic, theory , , , , , Leave a comment

I always confuses on the definition, and wiki did not have any summary. so,

The Original definition is the Hamiltonian of a magnetic dipole under external magnetic field \vec{B},

H = -\vec{\mu}\cdot \vec{B},

where \vec{\mu} is magnetic dipole moment (MDM). It is

\vec{\mu} = g \frac{q}{2 m} \vec{J} = g \frac{\mu}{\hbar} \vec{J} = \gamma \vec{J}.

Here, the g is the g-factor, \mu is magneton, and \vec{J} is the total spin, which has a intrinsic factor m\hbar / 2 inside. \gamma is gyromegnetic ratio.

We can see, the g-factor depends on the motion or geometry of the MDM. For a point particle, the g-factor is exactly equal to 2. For a charged particle orbiting, the g-factor is 1.

Put everything into the Hamiltonian,

H = -\gamma \vec{J}\cdot \vec{B} = -\gamma J_z B = -\gamma \hbar \frac{m}{2} B [J],

Because energy is also equal E = \hbar f , thus, we can see the \gamma has unit of frequency over Tesla.

———————————–

Take electron as an example, the MDM is Bohr magneton \mu_{e} = e\hbar/(2m_e). The MDM is,

\vec{\mu_e} = g_e \frac{e}{2 m_e} \vec{S} = g_e \frac{\mu_e}{\hbar}\vec{S} = \gamma_e \vec{S}.

The magnitude of MDM is,

|\vec{\mu_e}|= g_e \frac{e}{2 m_e} \frac{\hbar}{2} = \gamma_e \frac{\hbar}{2} [JT^{-1}],

The gyromagnetic ratio is,

\gamma_e = g_e \frac{\mu_e}{\hbar} [rad s^{-1} T^{-1}].

Since using rad s^{-1} is not convenient for experiment. The gyromagnetic ratio usually divided by 2\pi,

\gamma_e = g_e \frac{\mu_e}{2\pi\hbar} [Hz T^{-1}].

————————————-

To evaluate the magnitude of  MDM of  single particle state, which has orbital angular momentum and spin, the total spin \vec{J} = \vec{L} + \vec{S}. However, the g-factor for \vec{L} is difference from that for \vec{S}. Thus, the MDM is not parallel to total spin. We have to use Landé Formula,

\left< JM|\vec{V}|JM'\right> = \frac{1}{J(J+1)} \left< JM|(\vec{J}\cdot\vec{V})|JM\right> \left<JM|\vec{J}|JM'\right>

or see wiki, sorry for my laziness.

The result is

g=g_L\frac{J(J+1)+L(L+1)-S(S+1)}{2J(J+1)}+g_S\frac{J(J+1)-L(L+1)+S(S+1)}{2J(J+1)}

For J = L \pm 1/2,

g = J(g_L \pm \frac{g_S-g_L}{2L+1})

Electromagnetic multi-pole moment

March 7, 2011

GoLuckyRyan Basic, Porperties, theory , , , , , , , , , 5 Comments

Electromagnetic multipole comes from the charge and current distribution of the nucleons.

Magnetic multipole in the nucleus has 2 origins, one is the spin of the nucleons, and another is the relative orbital motion of the nucleons.  the magnetic charge or monopoles either do not exist or are very small. the next one is the magnetic dipole, which causes by the current loop of protons.

Electric multipole is solely by the proton charge.

From electromagnetism, we knew that the multipole has  different radial properties, from the potential of the fields:

\displaystyle \Psi(r) = \frac{1}{4\pi\epsilon_0} \int\frac{\rho(r')}{|r-r'|}d^3r'

\displaystyle A(r) = \frac{\mu_0}{4\pi}\int\frac{J(r')}{|r-r'|}d^3r'

and expand them into spherical harmonic by using:

\displaystyle \frac{1}{|r-r'|} = 4\pi\sum_{l=0}^{\infty}\sum_{m=-l}^{m=l} \frac{1}{2l+1}\frac{r_{<}^l}{r_>^{l+1}} Y_{lm}^*(\theta',\phi')Y_{lm}(\theta,\phi)

we have

\displaystyle \Psi(r) = \frac{1}{\epsilon_0} \sum_{l,m}\frac{1}{2l+1}\int Y_{lm}^*(\theta',\phi') r'^l\rho(r')d^3r' \frac{Y_{lm}(\theta,\phi)}{r^{l+1}}

\displaystyle A(r)=\mu_0 \sum_{l,m}\frac{1}{2l+1}\int Y_{lm}^*(\theta',\phi') r'^l J(r') d^3r' \frac{Y_{lm}(\theta,\phi)}{r^{l+1}}

we can see the integral gives us the required multipole moment. the magnetic and electric are just different by the charge density and the current density. we summarize in this way :

\displaystyle q_{lm} = \int Y^*_{lm}(\theta',\phi') r'^l \O(r') d^3 r'

where O can be either charge or current density. The l determines the order of multipole. and the potential will be simplified :

\displaystyle M(r)=\sum_{l,m}\frac{1}{2l+1} q_{lm} \frac{Y_{lm}(\theta,\phi)}{r^{l+1}}

where M can be either electric or magnetic potential, and I dropped the constant. since the field is given by 1st derivative, thus we have:

  1. monopole has 1/r^2 dependence
  2. dipole has 1/r^3
  3. quadrapole has 1/r^4
  4. and so on

The above radial dependences are the same for electric or magnetic. for an easy name of the multipole, we use L-pole, in which L can be 0 for the monopole, 1 for the dipole, 2 for the quadrupole, etc.. and we use E0 for the electric monopole, M0 for the magnetic monopole.

Since the nucleus must preserve parity, the parity for the electric and magnetic moment is diffident. the difference comes from the charge density and current density have different parity. The parity for charge density is even, but the current density is odd. 1/r^2 has even parity, 1/r^3 has odd parity. therefore

  • electric L-pole — (-1)^{L}
  • magnetic L-pole — (-1)^{L+1}

for easy comparison:

  • E0, E2, E4… and M1,M3, M5 … are even
  • E1,E3,E5…. and M0, M2, M4…. are odd

The expectation value for L-pole, we have to calculate :

\displaystyle \int \psi^* Q_{lm} \psi dx

where Q_{lm} is a multipole operator ( which is NOT q_{lm}), and its parity is follow the same rule. the parity of the wave function will be canceled out due to the square of itself. thus, only even parity are non-Zero. those are:

  • E0, E2, E4…
  • M1,M3, M5 …

that makes sense, think about a proton orbits in a circular loop, which is the case for E1, in time-average, the dipole momentum should be zero.

a review on Hydrogen’s atomic structure

February 2, 2011

GoLuckyRyan advance, atomic, Basic, review, theory , , , , , , , , , , , , , , , , , , Leave a comment

I found that most of the book only talk part of it or present it separately. Now, I am going to treat it at 1 place. And I will give numerical value as well. the following context is on SI unit.

a very central idea when writing down the state quantum number is, is it a good quantum number? a good quantum number means that its operator commute with the Hamiltonian. and the eigenstate states are stationary or the invariant of motion. the prove on the commutation relation will be on some post later. i don’t want to make this post too long, and with hyperlink, it is more reader-friendly. since somebody may like to go deeper, down to the cornerstone.  but some may like to have a general review.

the Hamiltonian of a isolated hydrogen atom is given by fews terms, deceasing by their strength.

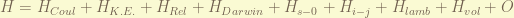
H = H_{Coul} + H_{K.E.} + H_{Rel} + H_{Darwin} + H_{s-0} + H_{i-j} + H_{lamb} + H_{vol} + O

the Hamiltonian can be separated into 3 classes.

__________________________________________________________

Bohr model

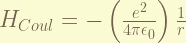
H_{Coul} = - \left(\frac {e^2}{4 \pi \epsilon_0} \right) \frac {1}{r}

is the Coulomb potential, which dominate the energy. recalled that the ground state energy is -13.6 eV. and it is equal to half of the Coulomb potential energy, thus, the energy is about 27.2 eV, for ground state.

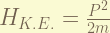
H_{K.E.} = \frac {P^2}{ 2 m}

is the non-relativistic kinetic energy, it magnitude is half of the Coulomb potential, so, it is 13.6 eV, for ground state.

comment on this level

this 2 terms are consider in the Bohr model, the quantum number, which describe the state of the quantum state, are

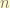n = principle number. the energy level.

l = orbital angular momentum. this give the degeneracy of each energy level.

m_l = magnetic angular momentum.

it is reasonable to have 3 parameters to describe a state of electron. each parameter gives 1 degree of freedom. and a electron in space have 3. thus, change of basis will not change the degree of freedom. The mathematic for these are good quantum number and the eigenstate \left| n, l, m_l \right> is invariant of motion, will be explain in later post. But it is very easy to understand why the angular momentum is invariant, since the electron is under a central force, no torque on it. and the magnetic angular momentum is an invariant can also been understood by there is no magnetic field.

the principle quantum number n is an invariance. because it is the eigenstate state of the principle Hamiltonian( the total Hamiltonian )!

the center of mass also introduced to make more correct result prediction on energy level. but it is just minor and not much new physics in it.

Fine structure

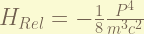
H_{Rel} = - \frac{1}{8} \frac{P^4}{m^3 c^2}

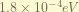is the 1st order correction of the relativistic kinetic energy. from K.E. = E - mc^2 = \sqrt { p^2 c^2 + m^2c^4} - mc^2 , the zero-order term is the non-relativistic kinetic energy. the 1st order therm is the in here. the magnitude is about 1.8 \times 10^{-4} eV . ( the order has to be recalculate, i think i am wrong. )

H_{Darwin} = \frac{\hbar^{2}}{8m_{e}^{2}c^{2}}4\pi\left(\frac{Ze^2}{4\pi \epsilon_{0}}\right)\delta^{3}\left(\vec r\right)

is the Darwin-term. this term is result from the zitterbewegung, or rapid quantum oscillations of the electron. it is interesting that this term only affect the S-orbit. To understand it require Quantization of electromagnetic field, which i don’t know. the magnitude of this term is about 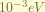10^{-3} eV

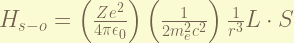
H_{s-o} = \left(\frac{Ze^2}{4\pi \epsilon_{0}}\right)\left(\frac{1}{2m_{e}^{2}c^{2}}\right)\frac{1}{r^3} L \cdot S

is the Spin-Orbital coupling term. this express the magnetic field generated by the proton while it orbiting around the electron when taking electron’s moving frame. the magnitude of this term is about 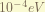10^{-4} eV

comment on this level

this fine structure was explained by P.M.Dirac on the Dirac equation. The Dirac equation found that the spin was automatically come out due to special relativistic effect. the quantum number in this stage are

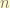n = principle quantum number does not affected.

l = orbital angular momentum.

m_l = magnetic total angular momentum.

s = spin angular momentum. since s is always half for electron, we usually omit it. since it does not give any degree of freedom.

m_s = magnetic total angular momentum.

at this stage, the state can be stated by \left| n, l, m_l, m_s \right> , which shown all the degree of freedom an electron can possible have.

However, 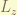L_z is no longer a good quantum number. it does not commute with the Hamiltonian. so, m_l does not be the eigenstate anymore. the total angular momentum was introduced J = L + S . and J^2 and J_z commute with the Hamiltonian.  therefore,

j = total angular momentum.

m_j = magnetic total angular momentum.

an eigenstate can be stated as 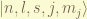\left| n, l, s, j, m_j \right> . in spectroscopy, we denote it as 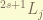^{2 s+1} L _j , where L is the spectroscopy notation for l .

there are 5 degrees of freedom, but in fact, s always half, so, there are only 4 real degree of freedom, which is imposed by the spin ( can up and down).  the reason for stating the s in the eigenstate is for general discussion. when there are 2 electrons, s can be different and this is 1 degree of freedom.

Hyperfine Structure

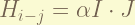
H_{i-j} = \alpha I \cdot J

is the nuclear spin- electron total angular momentum coupling. the coefficient of this term, i don’t know. Sorry. the nuclear has spin, and this spin react with the magnetic field generate by the electron. the magnitude is 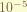10^{-5}

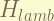
H_{lamb}

is the lamb shift, which also only affect the S-orbit.the magnitude is 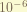10^{-6}

comment on this level

the hyperfine structure always makes alot questions in my mind. the immediate question is why not separate the orbital angular momentum and the electron spin angular momentum? why they first combined together, then interact with the nuclear spin?

may be i open another post to talk about.

The quantum number are:

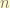n = principle quantum number

l = orbital angular momentum

s = electron spin angular momentum.

j = spin-orbital angular momentum of electron.

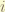i = nuclear spin. for hydrogen, it is half.

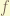f = total angular momentum

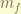m_f = total magnetic angular momentum

a quantum state is $\left| n, l, s, j,i, f , m_f \right>$. but since the s and i are always a half. so, the total degree of freedom will be 5. the nuclear spin added 1 on it.

Smaller Structure

H_{vol}

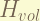
this term is for the volume shift. the magnitude is 10^{-10} .

in diagram:

Larmor Precession (quick)

January 23, 2011

GoLuckyRyan Spherical Stuffs, theory , , , , , , , , , , , , , , , 1 Comment

Magnetic moment (\mu ) :

this is a magnet by angular momentum of charge or spin. its value is:

\mu = \gamma J

where J is angular momentum, and \gamma is the gyromagnetic rato

\gamma = g \mu_B

Notice that we are using natural unit.

the g is the g-factor is a dimensionless number, which reflect the environment of the spin, for orbital angular momentum, g = 1.

\mu_B is Bohr magneton, which is equal to

\mu_B = \frac {e} {2 m} for positron

since different particle has different mass, their Bohr magneton value are different. electron is the lightest particle, so, it has largest value on Bohr magneton.

Larmor frequency:

When applied a magnetic field on a magnetic moment, the field will cause the moment precess around the axis of the field. the precession frequency is called Larmor frequency.

the precession can be understood in classical way or QM way.

Classical way:

the change of angular momentum is equal to the applied torque. and the torque is equal to the magnetic moment  cross product with the magnetic field. when in classical frame, the angular momentum, magnetic moment, and magnetic field are ordinary vector.

\vec {\Gamma}= \frac { d \vec{J}}{dt} = \vec{\mu} \times \vec{B} = \gamma \vec {J} \times \vec{B}

solving gives the procession frequency is :

\omega = - \gamma B

the minus sign is very important, it indicated that the J is precessing by right hand rule when \omega >0 .

QM way:

The Tim dependent Schrödinger equation (TDSE) is :

i \frac {d}{d t} \left| \Psi\right> = H \left|\Psi\right>

H is the Hamiltonian, for the magnetic field is pointing along the z-axis.

H = -\mu \cdot B = - \gamma J\cdot B = -gamma B J_z = \omega J_z

the solution is

\left|\Psi(t) \right> = Exp( - i \omega t J_z) \left| \Psi(0) \right>

Thus, in QM point of view, the state does not “rotate” but only a phase change.

However, the rotation operator on z-axis is

R_z ( \theta ) = Exp( - i \frac {\theta}{\hbar} J_z )

Thus, the solution can be rewritten as:

\left|\Psi (t)\right> = R_z( \omega t) \left|\Psi(0)\right>

That makes great analogy on rotation on a real vector.